
|
La Terre n'est pas un point, mais a une dimension finie. Or l'intensité du champ gravitationnel de la Lune varie comme l'inverse du carré de la distance à la Lune. Il en résulte une attraction différentielle qui déforme la Terre.
On peut estimer la valeur du champ de marée 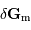 dans le cadre du modèle de l'océan global. On démontre que le module de
dans le cadre du modèle de l'océan global. On démontre que le module de 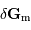 est de l'ordre de
est de l'ordre de 
Calcul du champ de marée. On estime la marée créée par la Lune  en un point courant
en un point courant  du globe terrestre, que l'on repère par rapport au
centre
du globe terrestre, que l'on repère par rapport au
centre 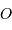 de la Terre.
On note
de la Terre.
On note 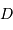 la distance
la distance 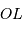 , et
, et 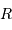 le rayon terrestre.
La composante du champ de marée
le rayon terrestre.
La composante du champ de marée 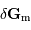 en
en  représente la différence du champ lunaire
entre les points
représente la différence du champ lunaire
entre les points 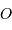 et
et 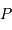 . Les calculs sont menés au 1er ordre par rapport au petit terme
. Les calculs sont menés au 1er ordre par rapport au petit terme 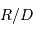 (car
(car 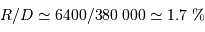 ) :
) :
![\begin{eqnarray*} \delta \mathbf{G} _{\mathrm{m}} &=& {{\cal G} m} { {\mathbf{PL}} \over PL^{3}} - {{\cal G} m} { {\mathbf{OL}} \over OL^{3}} \\ &=& {{\cal G} m} \left[{ {\mathbf{PO}}+ {\mathbf{OL}} \over PL^{3}} - { {\mathbf{OL}} \over OL^{3}} \right]\\ &\simeq& {{\cal G} m} \left[ { {\mathbf{PO}} \over {D}^{3}} + { {\mathbf{OL}} \over PL^{3}} - { {\mathbf{OL}} \over {D}^{3}} \right]\\ \end{eqnarray*}](../pages_maree-terrestre/equations_approche-statique/equation18.png)
On estime alors le terme 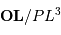 de l'équation précédente, en injectant la relation de Chasles
de l'équation précédente, en injectant la relation de Chasles 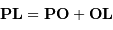 , et toujours au premier ordre en
, et toujours au premier ordre en 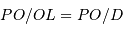 :
:
![\begin{eqnarray*} { {\mathbf{OL}} \over PL^{3}}&=& { {\mathbf{OL}} \over [{ {\mathbf{PL}}^{2}}]^{3/2}}= { {\mathbf{OL}} \over [( {\mathbf{PO}}+ {\mathbf{OL}})^{2}]^{3/2}}\\ \\ &\simeq& { {\mathbf{OL}} \over {D}^{3} \left( 1 + 2\ {\mathbf{PO}} . {\mathbf{OL}} / {D}^{2} \right)^{3/2}}\\ &\simeq& { {\mathbf{OL}} \over {D}^{3}} \left(1 - 3\ {\mathbf{PO}} . {\mathbf{OL}} / {D}^{2} \right)\\ \end{eqnarray*}](../pages_maree-terrestre/equations_approche-statique/equation22.png)
On trouve alors pour le champ de marée 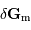 , en introduisant les
vecteurs unitaires
, en introduisant les
vecteurs unitaires 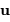 et
et  tels que
tels que 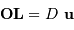 et
et 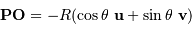 :
:
![\begin{eqnarray*} \delta \mathbf{G} _{\mathrm{m}} &=& {{\cal G} m} \left[ { {\mathbf{PO}} \over {D}^{3}} - {3\ {\mathbf{PO}} . {\mathbf{OL}} \over {D}^{2}}\ { {\mathbf{OL}} \over {D}^{3}} \right]\\ &=& {{\cal G} m\over {D}^{3}} \left[-R\ (\cos\theta\ \mathbf{u} + \sin\theta\ \mathbf{v}) + 3 R\ \cos\theta\ \mathbf{u} \right]\\ &=& {{\cal G} m R\over {D}^{3}} \left[2 \cos \theta\ \mathbf{u} - \sin \theta\ \mathbf{v} \right]\\ \end{eqnarray*}](../pages_maree-terrestre/equations_approche-statique/equation28.png)
On peut comparer les modules des champs de marée et gravitationnel :
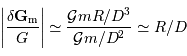
Il ressort de cette analyse que l'effet de marée :